Current Mirror and Bandgap

This article intends to introduce the basic concepts of current mirrors and the bandgap reference.

Basic Current Mirrors

Shortcomings of voltage biasing:

• Sensitive to threshold voltage variation
• Temperature dependence
• Rely on the precision of $V_{DD}$

Use of current for biasing may eliminate those problems. Use of identical MOS operating in saturation may suffice.

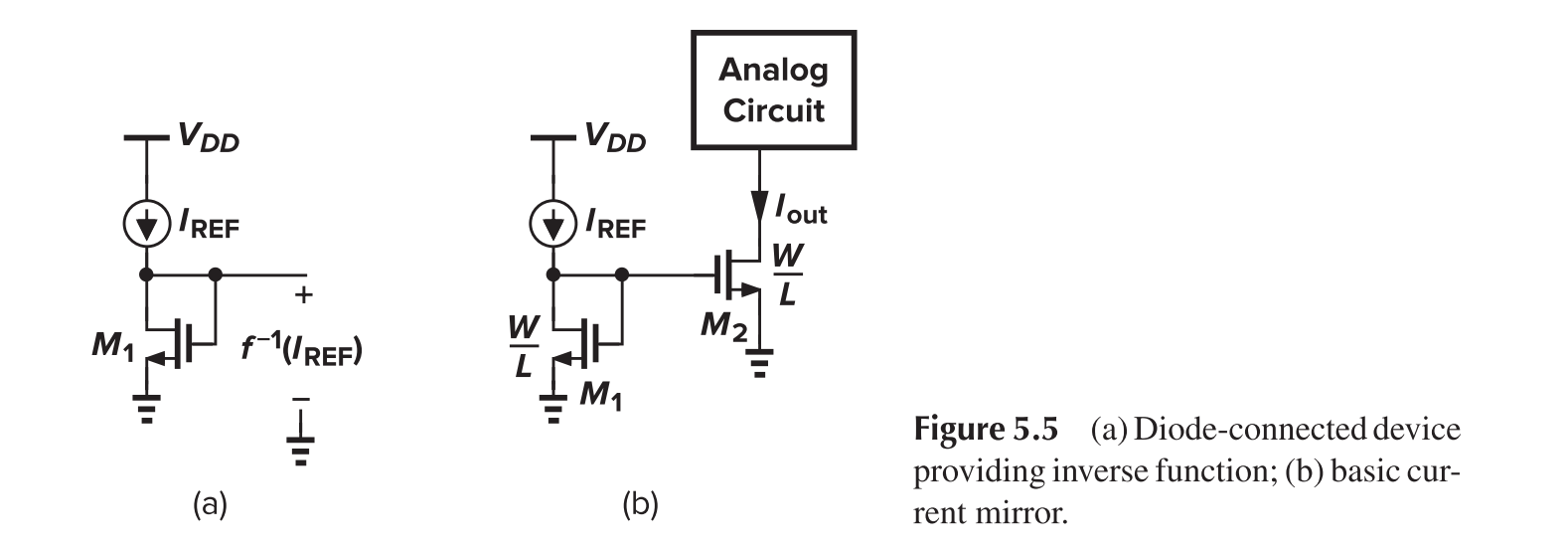
Here we have

$$I_{REF}=\frac{1}{2}\mu_nC_{ox}\left(\frac{W}{L}\right)_1(V_{GS}-V_{TH})^2\\ I_{out}=\frac{1}{2}\mu_nC_{ox}\left(\frac{W}{L}\right)_2(V_{GS}-V_{TH})^2$$

and thus

$$I_{out}=\frac{(W/L)_2}{(W/L)_1}I_{REF}$$

The transistor on the left generate $V_{GS}$ from $I_{REF}$ when and only when it is configured as a diode.

Note that:

• Mirror transistor should have the same length as the operating transistor to minimize mismatch.
• Mirror transistor should have the same width as the operating transistor to reduce errors caused by the poorly defined gate sides (Number of fingers and devices may be changed).

We can connect current mirrors in series as to modify the equivalent length of the transistor as follows:

Cascaded Current Mirror

If we consider channel-length modulation, the equations may be rewritten as

$$I_{REF}=\frac{1}{2}\mu_nC_{ox}\left(\frac{W}{L}\right)_1(V_{GS}-V_{TH})^2(1+\lambda V_{DS1})\\ I_{out}=\frac{1}{2}\mu_nC_{ox}\left(\frac{W}{L}\right)_2(V_{GS}-V_{TH})^2(1+\lambda V_{DS2})$$

and thus

$$I_{out}=\frac{(W/L)_2}{(W/L)_1}\cdot \frac{1+\lambda V_{DS2}}{1+\lambda V_{DS1}}I_{REF}$$

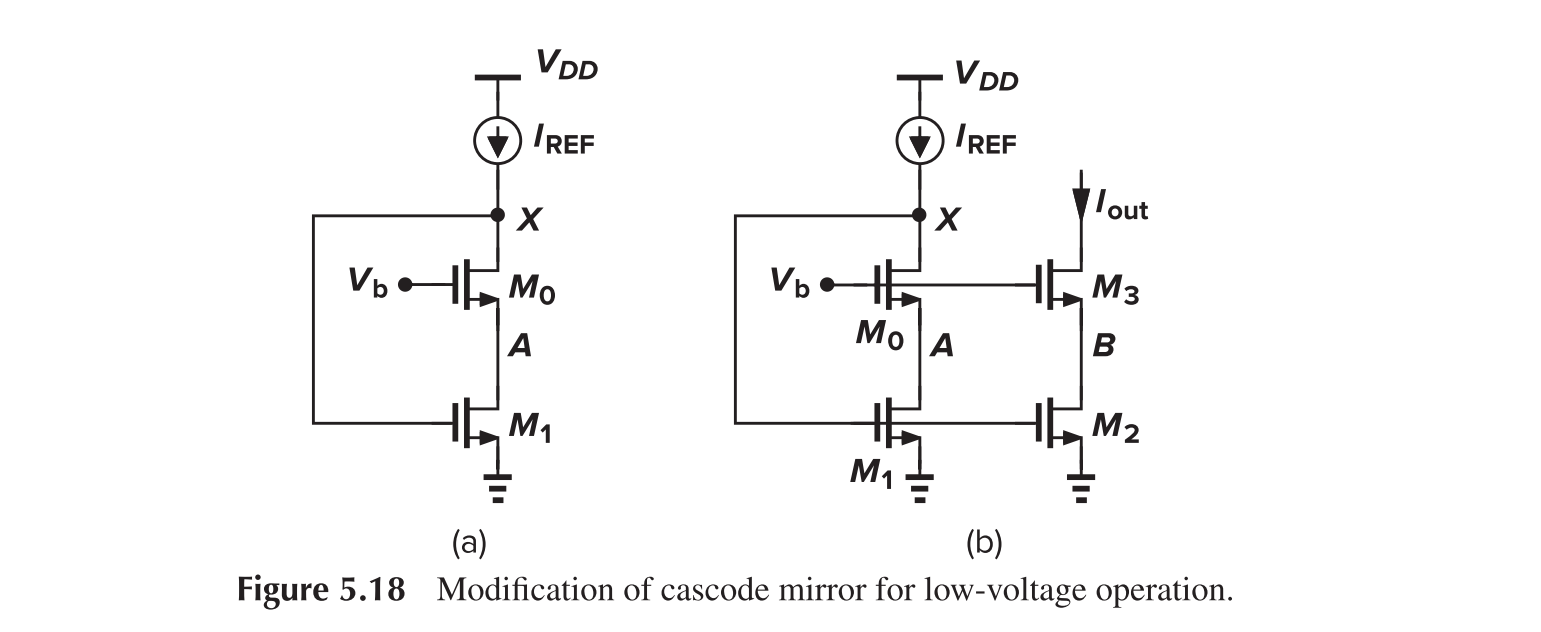
while $V_{DS1}=V_{GS1}=V_{GS2}$, $V_{DS1}$ may not equal to $V_{DS2}$. We may introduce the Cascode structure to shield the variation at node Y from that at node P

Approach 1

We need

$$V_{DS2}=V_{GS2}$$

and thus

$$V_b=V_{GS3}+V_{DS2}=V_{GS3}+V_{GS2}=V_{GS3}+V_{GS1}$$

We surmise that $V_b$ can be achieved by cascading two current sources, as in (c), as long as

$$V_{GS0}+V_{GS1}=V_{GS3}+V_{GS1}$$

or

$$V_{GS0}=V_{GS3}$$

Generally, we have

• $L_0=L_3$ to the lowest possible value to save area
• $W_3/W_0=W_2/W_1$ for $V_{GS0}=V_{GS3}$
• $L_1=L_2$ may be longer to reduce channel-length modulation and the flicker noise

The minimal allowable voltage at Node P is two overdrive voltage plus one threshold voltage.

Approach 2

In order to save voltage headroom, we may set $V_b$ to a lower value of

$$V_b=V_{DS2}+V_{GS3}=V_{GS2}-V_{TH1}+V_{GS1}$$

And therefore, we need to reduce $V_{DS1}$ to the value of $V_{DS2}$. A resistor is added to cause such a voltage drop

$$V_{TH}=I_{REF}R_1$$

Definition of $V_b$ may be realized as in figure (c), where

$$\begin{aligned} V_{GS5}=V_{GS3}&\\ V_{GS6}-R_6I_6&=V_{GS1}-V_{TH1}\\ &=V_{GS1}-R_1I_{IREF} \end{aligned}$$

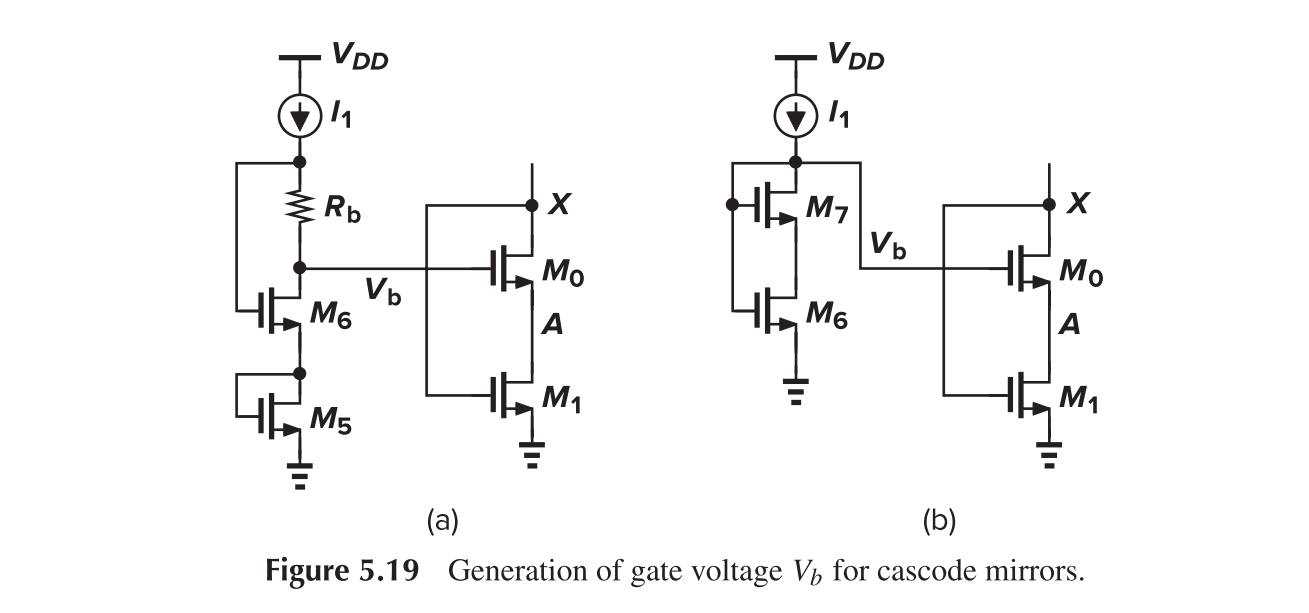
A further modification can be made to address the PVT problem

Here, $V_b$ can be chosen to place M1 at the edge of saturation. Note that if $V_{GS0}=V_{GS3}$, then $V_{DS2}$ is forced to be equal to $V_{DS1}$. The range of $V_b$ can be determined as

$$V_{GS1}-V_{TH1}\le V_b-V_{GS0}\\ V_{b}-V_{TH0}\le V_{GS1}$$

Hence $V_{GS0}-V_{TH0}<V_{TH1}$, and M0 should be large enough. Two methods can be used to generate $V_b$

Active Current Mirror

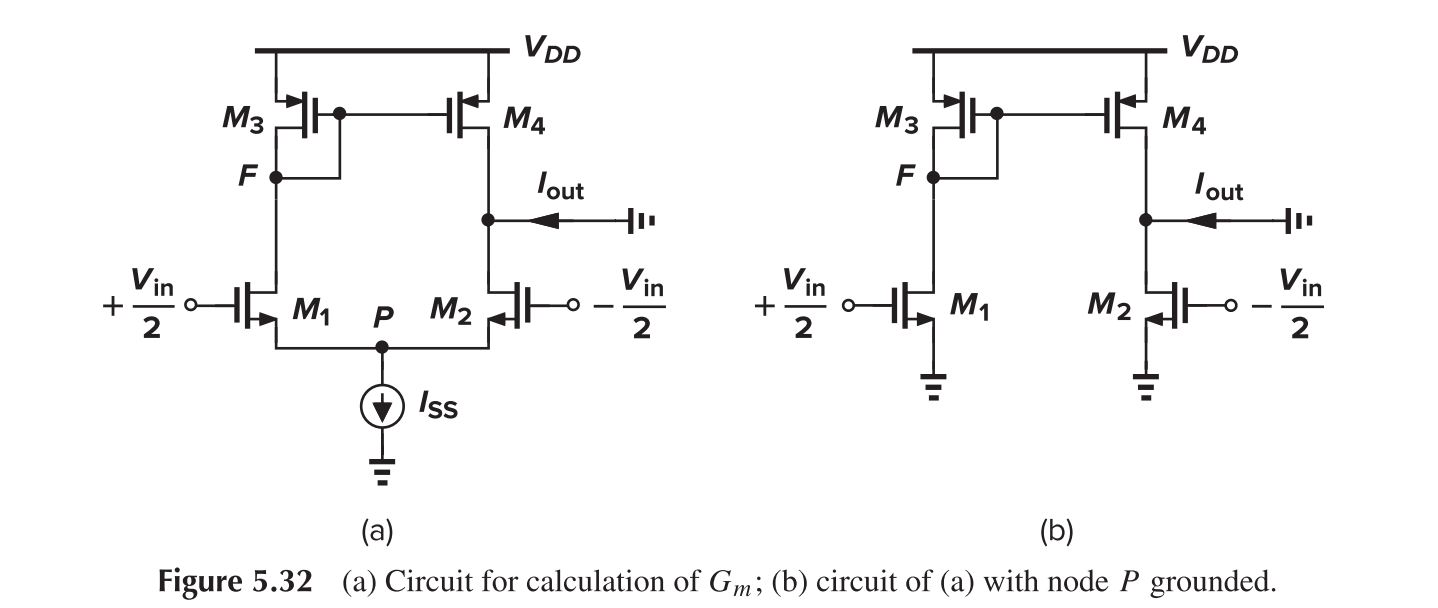
We have

$$\begin{aligned} G_m&=-g_{m1}r_{o1}\frac{g_{m4}r_d+1}{r_d+2r_{o1}}\\ &\approx g_m\\ R_{out}&=\frac{(1+g_{m4}r_d)r_{o4}+2r_{o1}+r_d}{(2r_{o1}+r_d)r_{o4}}\\ &\approx r_{o2}||r_{o4}\\\\ r_d&=r_{o3}||(1/g_{m3}) \end{aligned}$$

Supply-Independent Biasing

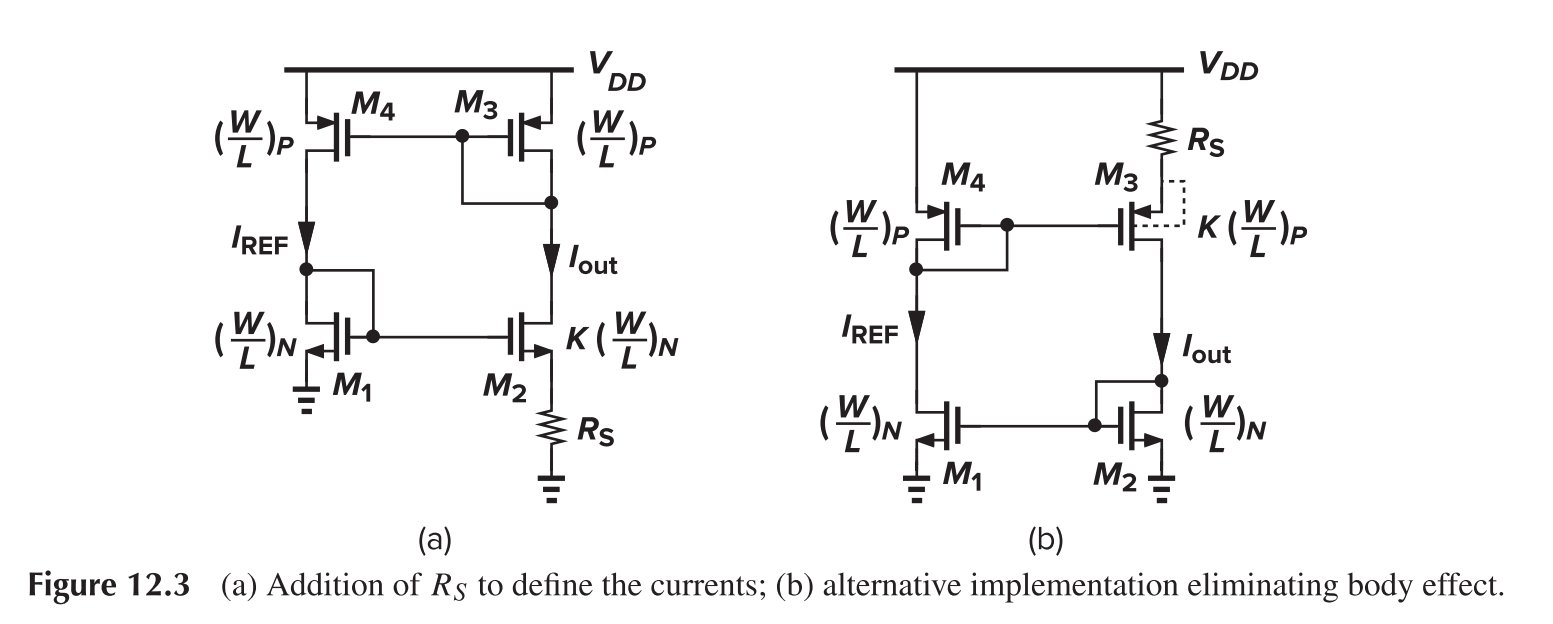
For (a)

$$\begin{aligned} &\sqrt{\frac{2I_{out}}{\mu_nC_{ox}(W/L)_N}}+V_{TH1}\\=&\sqrt{\frac{2I_{out}}{\mu_nC_{ox}K(W/L)_N}}+V_{TH2}+I_{out}R_s \end{aligned}$$

and hence

$$I_{out}=\frac{2}{\mu_nC_{ox}(W/L)_N}\cdot\frac{1}{R_S^2}\left(1-\frac{1}{\sqrt{K}}\right)^2$$

Relatively long channels are used for all of the transistors in the circuit to avoid channel-length modulation. Another issue is the existence of "degenerate" bias point, where all transistors carry zero current.

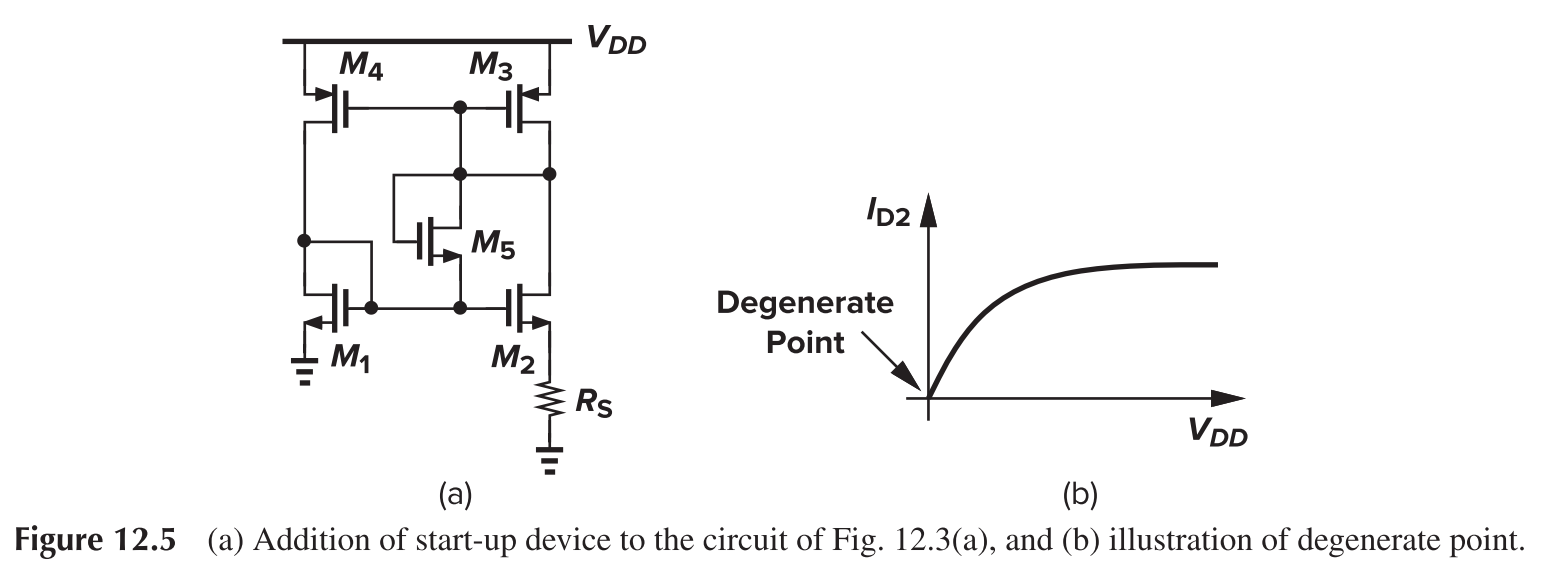
The “start-up” problem can be resolved by adding a mechanism that drives the circuit out of the degenerate bias point when the supply is turned on. Diode M3, M5, and M1 in series from VDD to GND could effectively prevent off states.

Temperature-Independent References

Negative-TC Voltage

For a bipolar device

$$\begin{aligned} I_C&=I_S\exp(\frac{V_{BE}}{V_T})\\ V_{BE}&=V_T\ln\frac{I_C}{I_S}\\ V_T&=\frac{kT}{q} &\text{Thermal Voltage}\\ I_S&\propto\mu kTn_i^2 &\text{Saturation Current}\\ \mu&\propto\mu_0T^m &\text{Minor Carrier Mobility}\\ n_i^2&\propto T^3\exp(-\frac{E_g}{kT}) &\text{Intrinsic Carrier Concentration}\\ E_g&\approx 1.12\text{eV} &\text{Bandgap Energy} \end{aligned}$$

We may derive (assume for now that $I_C$ is held constant)

$$\begin{aligned} \frac{\partial V_{BE}}{\partial T}&=\frac{\partial V_T}{\partial T}\ln\frac{I_C}{I_S}-\frac{V_T}{I_S}\frac{\partial I_S}{\partial T}\\ &=\frac{V_{BE}-(4+m)V_T-E_g/q}{T} \end{aligned}$$

Temperature coefficient of $V_{BE}$ itself depends on the temperature, creating error in constant reference generation if the positive-TC quantity exhibits a constant temperature coefficient.

Positive-TC Voltage

If two bipolar transistors operate at unequal current densities, then the difference between their base-emitter voltages is directly proportional to the absolute temperature.

$$\begin{aligned} \Delta V_{BE}&=V_{BE1}-V_{BE2}\\ &=V_T\ln\frac{nI_0}{I_{S1}}-V_T\ln\frac{I_0}{I_{S2}}\\ &=V_T\ln n\\ \frac{\partial \Delta V_{BE}}{\partial T}&=\frac{k}{q}\ln n \end{aligned}$$

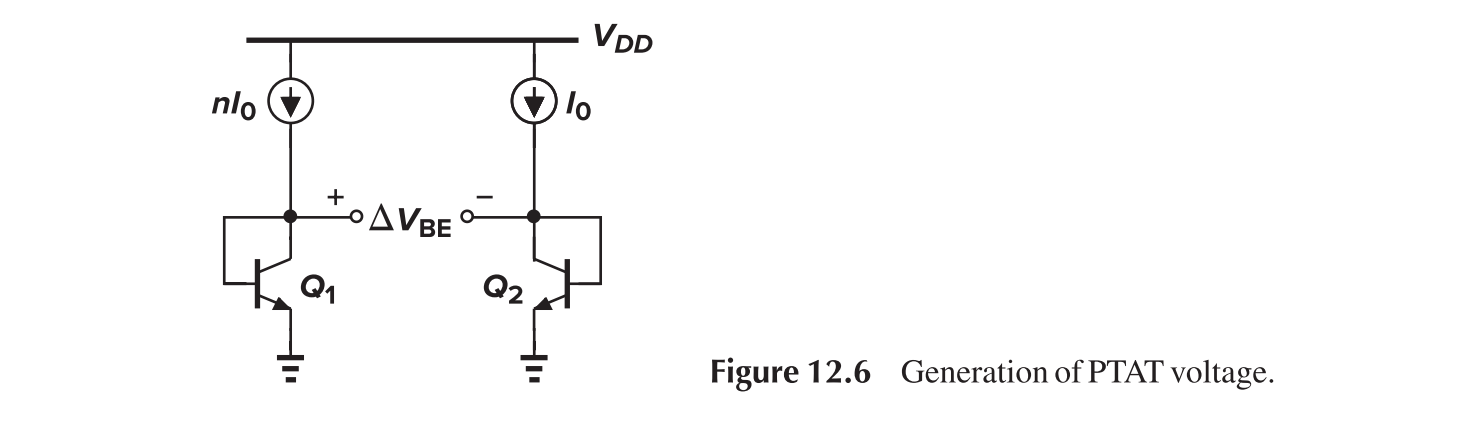
Bandgap Reference

Since at room temperature, $\partial V_{BE}/\partial T\approx−1.5\text{ mV/K}$ whereas $\partial V_{T}/\partial T\approx+0.087\text{ mV/K}$, hence $V_{REF}\approx V_{BE}+17.2V_T\approx1.25\text{ V}$.we can add the two as follows

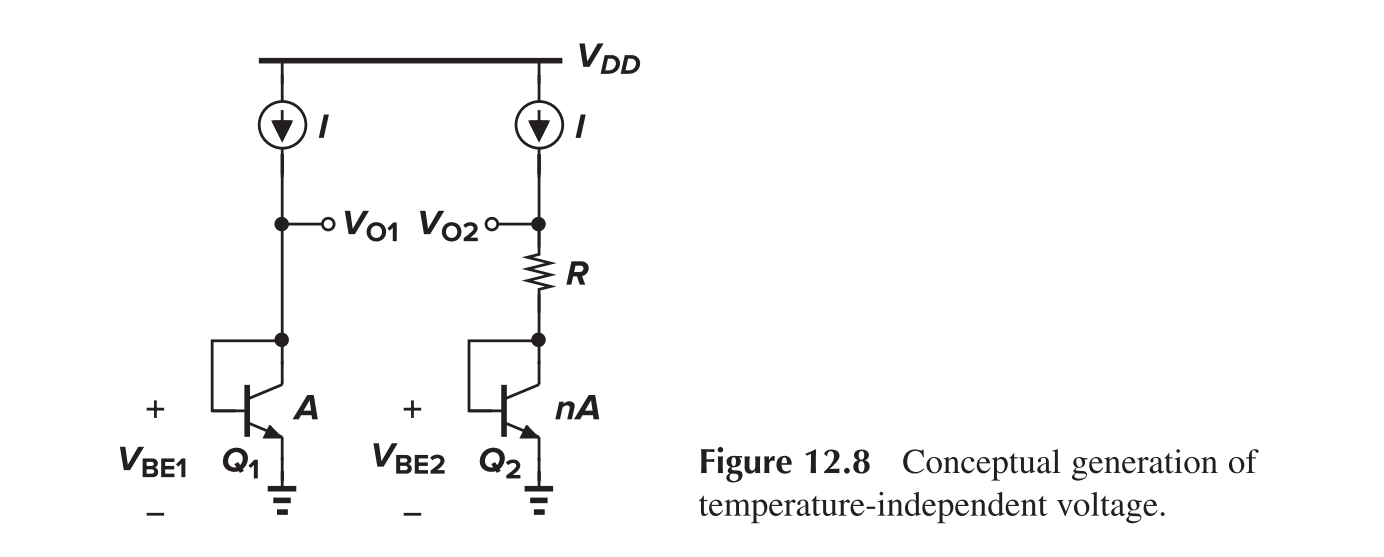
If we guarantee that $V_{O1}=V_{O2}$, then $RI=V_{BE1}-V_{BE2}=V_T\ln n$, and then $V_{O2}=V_{BE2}+V_T\ln n$. Yet, since $V_{O2}=V_{O1}\approx800\text{ mV}<1.25\text{ V}$, it cannot work as expected.

$$V_{out}=V_{BE2}+\frac{V_T\ln n}{R_3}(R_3+R_2)$$

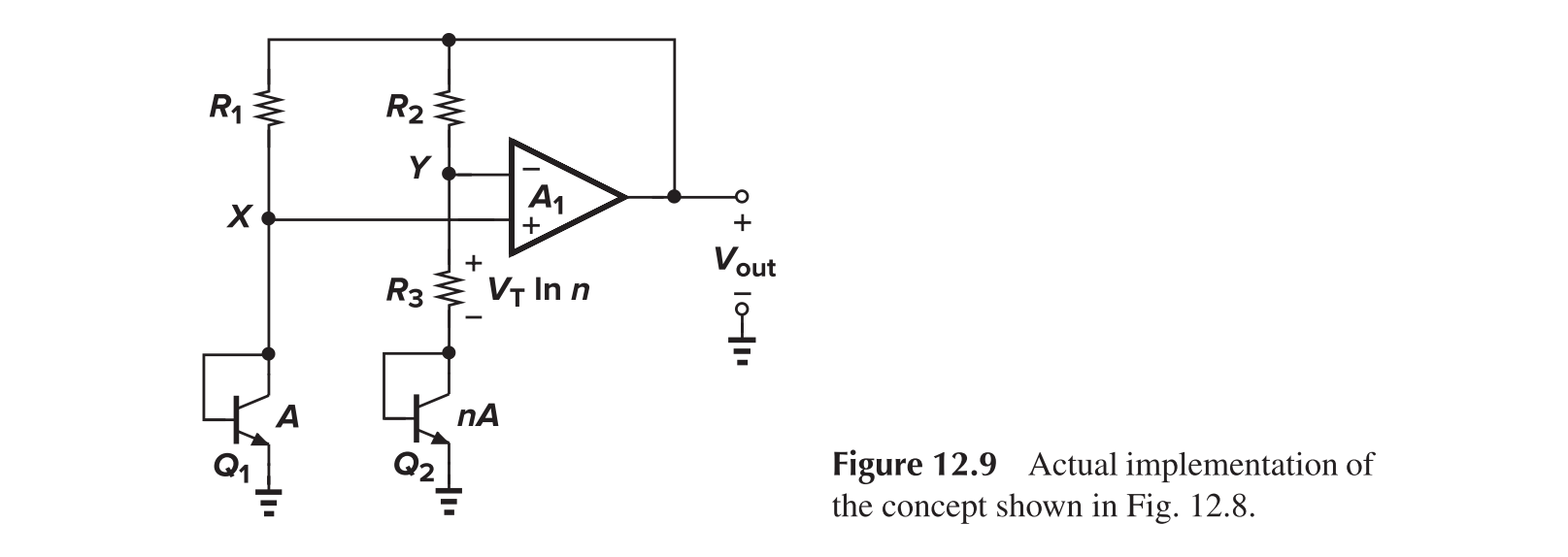
1. The op-amp ensures that $V_X=V_Y$
2. $R_1=R_2$ and $V_X=V_Y$ ensures that $I_1=I_2$
3. $V_Y$ is amplified before being added to $V_{BE2}$, hence voltage difference of $V_Y$ and $V_{O2}$ can be small